Contents
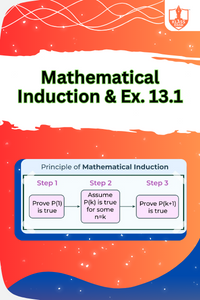
Mathematical Induction & Ex. 13.1
Description: Mathematical induction proves a statement for all natural numbers by showing it holds for a base case and a recursive step & Ex.13.1 Solutions.

Ex. 13.1 Part-II
Description: Ex. 13.1 Part-II Solutions

Ex. 13.1 Part-III
Description: Ex. 13.1 Part-III Solutions

Ex. 13.1 Part-IV, Positive Integral Index & Ex.13.2
Description: The binomial theorem expands expressions raised to a positive integral power, showing the coefficients of each term systematically & Ex.13.2 Solutions.

Ex. 13.2 Part-II
Description: Ex. 13.2 Part-II Solutions

General & Middle Term Expansion & Ex.13.3
Description: The general expansion of a binomial theorem computes any term, while the middle term expansion finds the term at the midpoint & Ex.13.3 Solutions.

Ex.13.3 Part -II
Description: Ex.13.3 Part -II Solutions

Ex.13.3 Part -III
Description: Ex.13.3 Part -III Solutions

Negative Index or Fraction & Ex. 13.4
Description: The Binomial Theorem for negative or fractional indices expands expressions into an infinite series, useful for approximations, and applies when the absolute value of x is less than one & Ex. 13.4 Solutions

Binomial Coefficients & Ex. 13.5
Description: Binomial coefficients represent the number of ways to choose k items from n, crucial in combinatorics, probability, and polynomial expansions & Ex. 13.5 Solutions.